Recursive ordinal

In mathematics, specifically set theory, an ordinal $\alpha$ is said to be recursive if there is a recursive binary relation $R$ that well-orders a subset of the natural numbers and the order type of that ordering is $\alpha$ .

It is trivial to check that 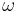$\omega$ is recursive, the successor of a recursive ordinal is recursive, and the set of all recursive ordinals is closed downwards. We call the supremum of all recursive ordinals the Church-Kleene ordinal and denote it by 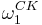$\omega^{CK}_1$ . Since the recursive relations are parameterized by the natural numbers, the recursive ordinals are also parameterized by the natural numbers. Therefore, there are only countably many recursive ordinals. Thus, 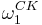$\omega^{CK}_1$ is countable.

The recursive ordinals are exactly the ordinals that have an ordinal notation in Kleene's 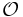$\mathcal{O}$ .

References

Rogers, H. The Theory of Recursive Functions and Effective Computability, 1967. Reprinted 1987, MIT Press, ISBN 0-262-68052-1 (paperback), ISBN 0-07-053522-1
Sacks, G. Higher Recursion Theory. Perspectives in mathematical logic, Springer-Verlag, 1990. ISBN 0-387-19305-7

Recursive ordinal

See also

References